Category Archives: MA 1505 Mathematics I

MA 1505 Mathematics I

Prediction of Final Exam 2014-2015 Semester I

Leave a comment

Module:                 MA 1505 Mathematics I

Time:                      2 hours ( 120 minutes ), Saturday, 22-Nov-2014 (Morning)

Questions:             8 questions, each question contains two questions. i.e. 16 questions.

Average speed:     7.5 minutes per question.

Scores:                  20% mid-term exam, 80% final exam. i.e. Each question in the final exam is 5%.

Remark:                 Another Possibility: 5 Chapters, each chapter contains 1 big question, and each question contains three small questions, i.e. 15 questions. 8 minutes per question.

The contents in high school:

Trigonometric functions, some basic inequalities and identities.

The contents before mid-term exam: Please review the details of them.

Chapter 2: Differentiation

Derivatives of one variable functions, derivatives of parameter functions, Chain rule of derivatives, the tangent line of the curve, L.Hospital Rule, critical points of one variable, local maximum and local minimum of one variable function.

Chapter 3: Integration

Integration by parts, Newton-Leibniz Formula, the area of the domain in the plane, the volume of the solid which is generated by a curve rotated with an axis.

Chapter 4: Series

Taylor Series and Power Series, radius of convergence of power series, the convergence domain of power series, the sum of geometric series and arithmetic series.

Chapter 5: Three Dimensional Spaces

Cross Product and Dot Product of vectors, projection of vectors, the equation of the plane and the line in 3-dimensional space, Distance from a point to a plane, Distance from a point to a line, the distance between two lines in two or three dimensional spaces, the distance between two parallel planes. Intersection points of two different curves.

The contents after mid-term exam: Must prepare them.

By the way, 2-3 questions means at least 2 questions, at most 3 questions. 0-1 question means 0 question or 1 question.

Geometric Graphs in Three Dimensional Space:

http://www.wolframalpha.com

z=x^{2}+y^{2}, z=-(x^{2}+y^{2})              infinite paraboloid

z=x^{2}-y^{2}             hyperbolic paraboloid

(x-x_{0})^{2}+(y-y_{0})^{2}+(z-z_{0})^{2}=R^{2}  sphere with radius R>0 and center (x_{0},y_{0},z_{0})

x^{2}+y^{2}=R^{2},  y^{2}+z^{2}=R^{2}, z^{2}+x^{2}=R^{2}       cylinder

ax+by+cz=d, \text{ where } a,b,c,d \in \mathbb{R}             Plane

y=x^{2}+c \text{ and } x=y^{2}+c, \text{ where } c\in \mathbb{R}             Parabola

Chapter 6: Fourier Series:

Fourier Coefficients of functions with period 2\pi: 1 question. Especially, a_{2014} and b_{2014}  (Integration by parts).

Fourier Coefficients of functions with period 2L: 1 question, where L is a positive real number. Especially, a_{2014} and b_{2014} (Integration by parts).

Calculate the summation of Fourier coefficients: 0-1 question. Especially, \sum_{n=0}^{\infty} a_{n} and \sum_{n=1}^{\infty} a_{n}.

Cosine and sine expansion of function on the half domain: 1 question.

Chapter 7: Function of Several Real Variables

Directional derivatives, partial derivatives, gradient of functions with two or three variables, Chain Rule of partial derivatives: 1-2 questions. (Pay attention to whether the vector is a unit vector or not. If it is not a unit vector, you should change it to a unit vector first, and then calculate the directional derivatives).

Critical points of two variable functions (saddle point, local maximum, local minimum): 0-1 question. (Calculate the partial derivatives first, then evaluate the critical points, so we can decide the property of the critical points from some rules).

Lagrange’s method: 0-1 question. (Calculate the maximum value of functions under some special conditions. Construct the function first, evaluate partial derivatives secondly, and calculate the critical points of the new functions. In addition, if you use  inequality “arithmetic mean” is greater than “geometric mean”, then the question will become easier.)

Chapter 8: Multiple Integral

Double integral, polar coordinate: 1 question. (The formula of polar coordinate in the plane).

Reverse the order of integration of double integral: 1 question. (Draw the picture of domain R and reverse the order of dx and dy).

Volume of the solid: 1 question. (Double integral, find the function z=z(x,y) and the domain R on the xy-plane. If the domain R is a disk or a sector, then you can use the polar coordinate).

Area of the surface: 1 question. (Partial Derivatives of functions with two variables, the domain R on the xy-plane. If the domain R is a disk or a sector, then you can use the polar coordinate. The area of a surface is a special case of the surface integral of a scalar field).

Triple integral: 0-1 question. (The method to calculate the triple integral is similar to double integral).

Chapter 9: Line Integrals

Length of a curve: 0-1 question. (Parameter equation of the curves. Length of a curve is a special case of line integral of a scalar field).

Line integrals of scalar fields: 1 question. (The equation of line segment, the equation of the circle with radius R, the length of vectors). Geometric meaning: the area of the wall along the curve.

Line integrals of vector fields: 1 question. (The equation of line segments, the equation of the circle with radius R, Dot product of vectors). Physical meaning: Work done.

Conservative vector fields and Newton-Leibniz formula of gradient vector fields: 0-1 question. (Definition of conservative vector field and its equivalent condition. When the value of a line integral of vector field is independent to the curve C, where C has the fixed initial point and the terminal point?).

Green’s Theorem: 1 question. (Two cases: the boundary is open; the boundary is closed. If the curve is open, you should close it by yourself.) Pay attention to the orientation, i.e. anticlockwise and left hand rule.

Chapter 10: Surface Integrals

Tangent plain of a surface: 0-1 question. (Partial derivatives, Cross product of two vectors, Normal vector of a plane)

Surface integrals of scalar fields: 1 question. (The equation of surface z=z(x,y) and the projection of the surface on the xy-plane, Cross product of vectors, the length of vectors. Change the surface integrals of scalar fields to double integrals).

Surface integrals of vector fields: 1 question. (The equation of surface z=z(x,y) and the projection of the surface on the xy-plane, Cross product and Dot product of vectors).

Stokes’ Theorem: 1 question. (This is a rule on line integrals of vector fields and surface integrals of vector fields. Remember the operator curl. Pay attention to the orientation of the curve on the boundary, i.e. the right hand rule).

Divergence Theorem: 0-1 question. (This is a rule on surface integrals of vector fields and triple integrals. Remember the operator div).

MA 1505 Mathematics I

Prediction of Middle Term Test

Leave a comment

Module:                 MA 1505 Mathematics I

Time:                     1 hours ( 60 minutes )

Questions:            10 Multiple Choice Questions.

Average speed:     6 minutes per question.

Scores:                  20% in final score.

The contents in high school:

Trigonometric functions, some basic inequalities and identities.

Questions in middle term test:

Question 1. Derivatives, Tangent line of a function, Intersection point of tangent line and x-axis, y-axis. Basic Rules of differentiation, Chain Rule.

Question 2. Critical points of a function, how to calculate the maximum and minimum value of a function.

Question 3. Integration by parts, integrate trigonometric functions.

Question 4. Fundamental theorem of calculus.

Question 5. Find the area which is bounded by some curves.

Question 6. Mathematical models. ( e.g. light and ball drop, ship and so on).

Question 7. Radius of convergence of a power series, the interval of convergence of a power series.

Question 8. Calculate the Taylor series of functions, Calculate the coefficients of Taylor series.

Question 9. How  to use Taylor series to calculate the solution of an equation.

Question 10. How to use Taylor series to calculate the summation of some series. ( Integration and differentiation).

Question 11. The length of a curve, the tangent line of a curve.

Question 12. Dot product and cross product of two vectors, equation of planes, normal vector of a plane, distance between a point and a plane.

MA 1505 Mathematics I

MA 1505 Tutorial 1: Derivative

Leave a comment

Definition of Derivative:

f^{'}(x)=\lim_{\Delta x\rightarrow 0} \frac{f(x+\Delta x)-f(x)}{\Delta x}

Rule: Assume f(x) and g(x) are two differentiable functions, the basic rules of derivative are

(f\pm g)^{'}(x)=f^{'}(x)\pm g^{'}(x)

(f\cdot g)^{'}(x)= f^{'}(x) g(x) + f(x)g^{'}(x)

(f/g)^{'}(x)=(f^{'}(x)g(x)-f(x)g^{'}(x))/(g(x))^{2}

(f\circ g)^{'}(x)=f^{'}(g(x))g^{'}(x)

Definition of Critical Point: x_{0} is called a critical point of f(x), if f^{'}(x_{0})=0.

If f^{'}(x)>0 on some interval I, then f(x) is increasing on the interval I. Similarly, if f^{'}(x)<0 on some interval I, then f(x) is decreasing on the interval I.

Tangent Line: Assume f(x) is a differentiable function on the interval I, then the tangent line of f(x) at the point x_{0}\in I is y-f(x_{0})=f^{'}(x_{0})(x-x_{0}), where f^{'}(x_{0}) is the slope of the tangent line.

Derivative of Parameter Functions: Assume y=y(t) and x=x(t), the derivative y^{'}(x) is y^{'}(t)/x^{'}(t), because the Chain Rule of derivatives.

Question 1. Calculate the tangent line of the curve x^{\frac{1}{4}} + y^{\frac{1}{4}}=4 at the point (16,16).

Method (i). Take the derivative of the equation x^{\frac{1}{4}}+y^{\frac{1}{4}}=4 at the both sides, we get

\frac{1}{4}x^{-\frac{3}{4}} + \frac{1}{4}y^{-\frac{3}{4}} y^{'}=0.

Assume x=y=16, we have the derivative y^{'}(16)=-1. That means the tangent line of the curve at the point (16,16) is y-16=-(x-16). i.e. y=-x+32.

Method (ii). From the equation, we know y(x)=(4-x^{\frac{1}{4}})^{4} , then calculating the derivative directly. i.e.

y^{'}(x)=4(4-x^{\frac{1}{4}})^{3}\cdot (-1)\cdot \frac{1}{4}x^{-\frac{3}{4}}

Therefore, y^{'}(16)=-1.

Method (iii). Making the substitution x=4^{4}\cos^{8}\theta, y=4^{4}\sin^{8}\theta, then (16,16) corresponds to \theta=\pi/4. From the derivative of the parameter functions, we know

\frac{dy}{dx}= \frac{dy/d\theta}{dx/d\theta}=\frac{4^{4}\cdot 8\sin^{7}\theta\cdot \cos\theta}{4^{4}\cdot 8\cos^{7}\theta\cdot (-\sin\theta)}

If we assume \theta=\pi/4, then y^{'}(16)=-1.

Method (iv). Geometric Intuition. Since the equation x^{\frac{1}{4}}+y^{\frac{1}{4}}=4 is a symmetric graph with the line y=x, and (16,16) is also on the symmetric line. Therefore, the slope of the curve at the point (16,16) is -1. Hence, the tangent line is y=-x+32.

Question 2. Let y=(1+x^{2})^{-2} and x=\cot \theta. Find dy/dx and express your answer in terms of \theta.

Method (i). y=\frac{1}{1+x^{2}}= \sin^{2}\theta ,

\frac{dy}{dx}=\frac{dy/d\theta}{dx/d\theta} = \frac{2\sin\theta \cos \theta}{-\sin^{-2}\theta}= - \sin^{2}\theta\sin2\theta.

Method (ii). \frac{dy}{dx}=-\frac{2x}{(1+x^{2})^{2}} = -\frac{2\cot \theta}{(1+\cot^{2}\theta)^{2}}=-\sin^{2}\theta\sin 2\theta.

MA 1505 Mathematics I

Prediction of Final Exam 2013-2014 Semester I

Link 3 Comments

Module:                 MA 1505 Mathematics I

Time:                      2 hours ( 120 minutes )

Questions:             8 questions, each question contains two questions. i.e. 16 questions.

Average speed:     7.5 minutes per question.

Scores:                  20% mid-term exam, 80% final exam. i.e. Each question in the final              exam is 5%.

Remark:                 Another Possibility: 5 Chapters, each chapter contains 1 big question, and each question contains three small questions, i.e. 15 questions. 8 minutes per question.

The contents in high school:

Trigonometric functions, some basic inequalities and identities.

The contents before mid-term exam: Please review the details of them.

Chapter 2: Differentiation

Derivatives of one variable functions, derivatives of parameter functions, Chain rule of derivatives, the tangent line of the curve, L.Hospital Rule, critical points of one variable, local maximum and local minimum of one variable function.

Chapter 3: Integration

Integration by parts, Newton-Leibniz Formula, the area of the domain in the plane, the volume of the solid which is generated by a curve rotated with an axis.

Chapter 4: Series

Taylor Series and Power Series, radius of convergence of power series, the sum of geometric series and arithmetic series.

Chapter 5: Three Dimensional Spaces

Cross Product and Dot Product of vectors, projection of vectors, the equation of the plane and the line in 3-dimensional space, Distance from a point to a plane, Distance from a point to a line, the distance between two lines in two or three dimensional spaces, the distance between two parallel planes. Intersection points of two different curves.

The contents after mid-term exam: Must prepare them.

By the way, 2-3 questions means at least 2 questions, at most 3 questions. 0-1 question means 0 question or 1 question.

Geometric Graphs in Three Dimensional Space:

http://www.wolframalpha.com

z=x^{2}+y^{2}             infinite paraboloid

z=x^{2}-y^{2}             hyperbolic paraboloid

(x-x_{0})^{2}+(y-y_{0})^{2}+(z-z_{0})^{2}=R^{2}  sphere with radius R and center (x_{0},y_{0},z_{0})

x^{2}+y^{2}=R^{2}          cylinder

ax+by+cz=d, \text{ where } a,b,c,d \in \mathbb{R}             Plane

y=x^{2}+c \text{ and } x=y^{2}+c, \text{ where } c\in \mathbb{R}             Parabola

Chapter 6: Fourier Series:

Fourier series, Parseval’s identity: 2-3 questions. ( Integration by parts, calculate the sum of Fourier coefficients, period 2L functions ( where L is a positive real number), calculate the value of some special series from Fourier series, cosine expansion and sine expansion of function on the half domain).

Chapter 7: Multiple Variable Functions

Directional derivatives, partial derivatives, gradient of functions with two or three variables, Chain Rule of partial derivatives: 1-2 questions. (Pay attention to whether the vector is a unit vector or not. If it is not a unit vector, you should change it to a unit vector first, and then calculate the directional derivatives).

Critical points of two variable functions ( saddle point, local maximum, local minimum): 0-1 question. ( Calculate the partial derivatives first, then evaluate the critical points, so we can decide the property of the critical points from some rules).

Lagrange’s method: 0-1 question. ( Calculate the maximum value of functions under some special conditions. Construct the function first, evaluate partial derivatives secondly, and calculate the critical points of the new functions. In addition, if you use  inequality “arithmetic mean” is greater than “geometric mean”, then the question will become easier.)

Chapter 8: Multiple Integration

Double integration, polar coordinate: 1 question. ( The formula of polar coordinate in the plane).

Reverse the order of integration of double integration: 1 question. ( Draw the picture of domain R and reverse the order of dx and dy).

Volume of the solid: 1 question. ( Double integrals).

Area of the surface: 1 question. ( Partial Derivatives of two variable functions, Polar Coordinate).

Chapter 9: Line Integrals

Length of the curve: 0-1 question. ( Parameter equation of the curves).

Line integrals of scalar fields: 1 question. ( The equation of line segment, the equation of the circle with radius R, the length of vectors). Geometric meaning: the area of the wall along the curve.

Line integrals of vector fields: 1 question. ( The equation of line segments, the equation of the circle with radius R, Dot product of vectors). Physical meaning: Work done.

Conservative vector fields and Newton-Leibniz formula of gradient vector fields: 0-1 question. ( Definition of conservative vector field and its equivalent condition).

Green’s Theorem: 1 question. ( Two cases: the boundary is open; the boundary is closed. If the curve is open, you should close it by yourself.) Pay attention to the orientation, i.e. anticlockwise.

Chapter 10: Surface Integrals

Tangent plain of a surface: 0-1 question. ( Partial derivatives, Cross product of two vectors, Normal vector of a plane)

Surface integrals of scalar fields: 1 question. ( The equation of surface, Cross product of vectors, the length of vectors).

Surface integrals of vector fields: 1 question. ( The equation of surface, Cross product and Dot product of vectors).

Stokes’ Theorem: 1 question. ( Pay attention to the orientation).

Divergence Theorem: 0-1 question. ( Triple integrals).

MA 1505 Mathematics I

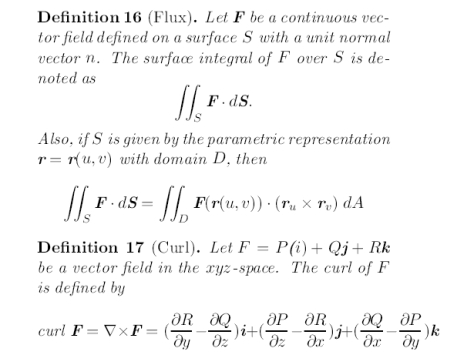
MA 1505 Tutorial 11: Surface Integral, Divergence Theorem and Stokes’ Theorem

Leave a comment

Surface Integrals of Scalar Fields: Assume f: U \subseteq \mathbb{R}^{3} \rightarrow \mathbb{R} is a function, r: D\subseteq \mathbb{R}^{2} \rightarrow \mathbb{R}^{3} is a surface S. Then the surface integral is

\iint_{S} f dS= \iint_{D} f(\textbf{r}(x,y)) || \textbf{r}_{x} \times \textbf{r}_{y} || dxdy

where the left hand side is the surface integral of the scalar field and the right hand side is the multiple integration. \textbf{r}_{x} \times \textbf{r}_{y} denotes the cross product between \textbf{r}_{x} and \textbf{r}_{y} ,

|| \textbf{r}_{x} \times \textbf{r}_{y} ||  denotes the length of the vector \textbf{r}_{x} \times \textbf{r}_{y}.

Remark.  If f(x,y,z)=1 for all (x,y,z) \in \mathbb{R}^{3} , and r: D\subseteq \mathbb{R}^{2} \rightarrow \mathbb{R}^{3} is a surface, then

the left hand side is \iint_{S} dS = \text{ the surface area of } S.

the right hand side is \iint_{D} \sqrt{1+(f_{x})^{2}+ (f_{y})^{2} } dxdy , since \textbf{r}(x,y)=(x,y,f(x,y)), \text{ where } (x,y) \in D, \textbf{r}_{x}=(1,0,f_{x}) and \textbf{r}_{y}=(0,1,f_{y}), the cross product \textbf{r}_{x} \times \textbf{r}_{y}= (-f_{x}, -f_{y},1).

That means:

\text{ the surface area of } S= \iint_{D} \sqrt{1+(f_{x})^{2}+(f_{y})^{2} }dxdy.

Surface Integrals of Vector Fields:

Imagine that we have a fluid flowing through S, such that \bold{F}(x) determines the velocity of the fluid at \bold{x}. The flux is defined as the quantity of fluid flowing through S per unit time.

This illustration implies that if the vector field is tangent to S at each point, then the flux is zero, because the fluid just flows in parallel to S, and neither in nor out. This also implies that if \bold{F} does not just flow along S, that is, if F has both a tangential and a normal component, then only the normal component contributes to the flux. Based on this reasoning, to find the flux, we need to take the dot product of \bold{F} with the unit normal vector to S at each point, which will give us a scalar field, and integrate the obtained field as above.

1280px-Surface_vectors

Assume \textbf{F} : U \subseteq \mathbb{R}^{3} \rightarrow \mathbb{R}^{3} is a vector field, r: D\subseteq \mathbb{R}^{2} \rightarrow \mathbb{R}^{3} is a surface S. Then the surface integrals of the vector field F is

\iint_{S} \textbf{F} \cdot d \textbf{S} = \iint_{S} \textbf{F} \cdot \textbf{n} dS

The left hand side is the surface integral of vector field and the right hand side is the surface integral of scalar function, since \textbf{F} \cdot \textbf{n} is a scalar function. That means,

\iint_{S} \textbf{F} \cdot d \textbf{S} = \iint_{S} \textbf{F} \cdot \textbf{n} dS = \iint_{D} \textbf{F}( \textbf{r}(x,y)) \cdot ( \textbf{r}_{x} \times \textbf{r}_{y}) dxdy

Divergence Theorem (Gauss’s theorem or Ostrogradsky’s theorem)

This theorem is a result that relates the flow (that is, flux) of a vector field through a surface to the behavior of the vector field inside the surface. More precisely, the divergence theorem states that the outward flux of a vector field through a closed surface is equal to the volume integral of the divergence over the region inside the surface. Intuitively, it states that the sum of all sources minus the sum of all sinks gives the net flow out of a region.

\iint_{S} \textbf{F} \cdot d \textbf{S} = \iiint_{V} \nabla \cdot \textbf{F} dV = \iiint_{V} (\frac{\partial P}{\partial x} + \frac{\partial Q}{\partial y} + \frac{\partial R}{\partial z}) dxdydz

where V \subseteq \mathbb{R}^{3} is a bounded domain and \partial V=S, \textbf{F}=(P,Q,R) is a vector field.

800px-Divergence_theorem.svg

Stokes’ Theorem

\int_{\partial \Sigma} \textbf{F} \cdot d\textbf{r} = \iint_{\Sigma} ( \textbf{curl F} ) \cdot d \textbf{S}

where \textbf{curl F}= (\frac{\partial R}{\partial y} - \frac{\partial Q}{\partial z}) \textbf{i} + (\frac{\partial P}{\partial z} - \frac{\partial R}{\partial x}) \textbf{j} + ( \frac{\partial Q}{\partial x}- \frac{\partial P}{\partial y}) \textbf{k} is a vector field. \Sigma is a compact surface and \partial \Sigma  is the boundary of \Sigma. The curve \partial\Sigma has the positive orientation, that means following the right hand rule.

429px-Stokes'_Theorem.svg

MA 1505 Mathematics I

MA 1505 Tutorial 9 and 10: Line Integral and Green’s Formula

Image Leave a comment

Line integral of a scalar field:

Assume f: U \subseteq \mathbb{R}^{n} \rightarrow \mathbb{R} is a smooth function,

where U is the domain of f.

\int_{C} f ds =\int_{a}^{b} f(r(t)) \cdot ||r^{'}(t)|| dt

where r:[a,b] \rightarrow C is a smooth curve.

Line integral of a vector field:

Assume \mathbf{F}: U \subseteq \mathbb{R}^{n} \rightarrow \mathbb{R}^{n} is a smooth vector function,

\int_{C} \mathbf{F} \cdot d\mathbf{r} = \int_{a}^{b} \mathbf{F}( \mathbf{r}(t)) \cdot \mathbf{r}^{'}(t) dt

where r:[a,b] \rightarrow C  is a smooth curve.

Green’s Formula: 

Assume \bold{F}=(P,Q) is a vector field,

\oint_{\partial D} \bold{F}\cdot d\bold{s}=\oint_{\partial D} Pdx + Qdy = \iint_{D} (\frac{\partial Q}{\partial x} - \frac{ \partial P}{\partial y}) dxdy,

where D is the domain and \partial D denotes the boundary of D. The orientation of \partial D satisfies the left hand rule. That means if you walk along the boundary of D, the domain D must be on your left.

429px-Green's-theorem-simple-region.svg

D is a simply connected region with boundary consisting four boundaries C_{1}, C_{2}, C_{3}, C_{4}, the orientation is counterclockwise.

macroscopic_microscopic_circulationmacroscopic_microscopic_circulation_hole

In the first graph, \partial D which denotes the boundary of D has only one closed curve C and the orientation of C is counterclockwise. However, in the second graph, \partial D contains two curves, i.e. the blue one and the red one. The orientation on the blue one which is the outer boundary of D is counterclockwise, the orientation on the red one which in the inner boundary of D is clockwise. That means if you walk along the boundary of D, the domain D must be on your left. This is the left hand rule.

Corollary of Green’s Theorem

Assume D is a domain in the plane, Area(D) denotes the area of D, then the area can be calculated from the following formulas:

Area(D)=\iint_{D} dxdy

= \oint_{\partial D} xdy = \oint_{\partial D} -ydx =\oint_{\partial D} (-\frac{y}{2} dx +\frac{x}{2} dy)

Fundamental Theorem of Line Integral:

\int_{C}\nabla f \cdot d\bold{r}=f(\text{terminal point})-f(\text{initial point}),

where C denotes a curve from initial point to terminal point and f is a scalar field.

Conservative Vector Field:

1. \bold{F}=(P,Q,R) is called a conservative vector field, if there exists a scalar field f such that \nabla f=\bold{F}. It is equivalent to these conditions:

\frac{\partial P}{\partial y}=\frac{\partial Q}{\partial x}, \frac{\partial Q}{\partial z}=\frac{\partial R}{\partial y}, \frac{\partial R}{\partial x}=\frac{\partial P}{\partial z}.

2. \bold{F}=(P,Q) is called a conservative vector field, if  there exists a scalar field f such that \nabla f=\bold{F}. It is equivalent the condition \frac{\partial P}{\partial y}=\frac{\partial Q}{\partial x}.

Path Independence:

A key property of a conservative vector field is that its integral along a path depends only on the endpoints of that path, not the particular route taken.

For example, if \bold{F}=(P,Q) or \bold{F}=(P,Q,R) is a conservative vector field, then the value of the line integral I=\int_{C} \bold{F}\cdot d\bold{r} depends only on the initial point and terminal point of the curve C. That means if \bold{F} is a conservative vector field, the curves C_{1} and C_{2} have the same initial points and terminal points, then these two line integrals are equal: \int_{C_{1}} \bold{F}\cdot d\bold{r}=\int_{C_{2}}\bold{F}\cdot d\bold{r}. For this reason, a line integral of a conservative vector field is called path independent.

Question 1. For each non-zero constant a>0, let C_{a} denote the curve y=a \sin x, where 0 \leq x \leq \pi. Let

I(a)= \int_{C_{a}} (1+y^{3})dx + (2x+y)dy

Find the minimum value of I(a) in the domain a>0.

Solution.

Method (i).  Use the definition of line integration.

Since y=a \sin x , 0 \leq x \leq \pi , dy= a \cos x dx ,

I(a)= \int_{0}^{\pi} (1+a^{3} \sin^{3} x) dx + (2x+ a \sin x) a \cos x dx

= \int_{0}^{\pi} ( 1+ a^{3} \sin^{3} x + 2a x \cos x + a^{2} \sin x \cos x) dx.

Since

\int_{0}^{\pi} (a^{3} \sin^{3} x) dx = \frac{4}{3} a^{3},

\int_{0}^{\pi} (2a x \cos x) dx =-4a,

\int_{0}^{\pi} (a^{2} \sin x \cos x) dx =0,

we get

I(a)= \pi + \frac{4}{3} a^{3} - 4a on a>0 .

I^{'}(a) = 4a^{2}-4 .

The minimum value is taken at a=1, the I(1)= \pi -\frac{8}{3}.

Method (ii). Use Green’s Formula.

Consider the domain D bounded by y= a\sin x and x=0, P(x,y)=1+y^{3} and Q(x,y)= 2x+y.

i.e.

D: 0\leq x \leq \pi, 0\leq y \leq a \sin x.

From Green’s Formula, pay attention to the orientation,

\iint_{D} ( 2-3y^{2}) dxdy = -I(a) + \int_{0}^{\pi} dx

Therefore,

I(a) = \pi - \iint_{D} ( 2- 3y^{2}) dxdy

= \pi - \int_{0}^{\pi} \int_{0}^{a \sin x} (2-3y^{2}) dy dx

= \pi - \int_{0}^{\pi} ( 2a \sin x - a^{3} \sin^{3} x) dx

= \pi - 4a + \frac{4}{3} a^{3}.

The derivative of I(a) is I^{'}(a) = 4 a^{2}-4, the minimum value is taken at a=1, and I(1)= \pi-\frac{8}{3}.

Question 2. Prove the area of the disc with radius R is \pi R^{2}.

Solution. 

Method (i). Definition of Integration.

Area=4 \int_{0}^{R} \sqrt{R^{2}-x^{2}} dx

= 4R^{2} \int_{0}^{\frac{\pi}{2}} cos^{2}\theta d \theta  (x=\sin \theta)

= 4R^{2} \int_{0}^{\frac{\pi}{2}} \frac{1+ \cos (2\theta)}{2} d\theta

= \pi R^{2}.

Method (ii). Green Formula

Area(D)=\iint_{D} dxdy

= \oint_{\partial D} xdy = \oint_{\partial D} -ydx =\oint_{\partial D} (-\frac{y}{2} dx +\frac{x}{2} dy)

For D=\{ x^{2}+y^{2}\leq R^{2} \}, on \partial D, x=\cos \theta, y=\sin\theta, where \theta \in [0, 2\pi).

Area(D)= \oint_{\partial D} x dy

= \int_{0}^{2\pi} R \cos \theta \cdot R \cos \theta d\theta

= \pi R^{2}.

Question 3. Prove the area of the ellipse \frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} =1 is \pi ab.

Solution.  It is similar to Question 2, and x=a \cos \theta, y= b \cos \theta.

Question 4.  Calculate

\int_{C} 2xy dx + (x^{2}+z)dy +y dz

where C consists two line segments: C_{1} from (0,0,0) to (1,0,2), and C_{2} from (1,0,2) to (3,4,1).

Solution. 

Method (i).  From the definition of line integral,

C_{1}: r_{1}(t)=(t,0,2t), C_{2}: r_{2}(t)=(1+2t, 4t, 2-t).

\int_{C_{1}} 2xy dx + (x^{2}+z)dy +y dz=0

\int_{C_{2}} 2xy dx + (x^{2}+z)dy +y dz

=\int_{0}^{1}( 48 t^{2} + 24 t +12) dt

=40.

Method (ii). Check the vector field \mathbf{F}=(2xy, x^{2}+z, y) is a conservative vector field. Since

\frac{\partial}{\partial y} (2xy)= \frac{\partial}{\partial x} (x^{2}+z)=2x

\frac{\partial}{\partial x}(y)=\frac{\partial}{\partial z}(2xy)=0

\frac{\partial}{\partial y}(y)=\frac{\partial}{\partial z}(x^{2}+z)=1

Therefore, \mathbf{F} is a conservative vector field, and we can assume \nabla f=\mathbf{F} , i.e. f_{x}=2xy, f_{y}=x^{2}+z, f_{z}=y. Hence,

f(x,y,z)=x^{2}y+yz+K for some constant K.

Therefore, the answer is f(3,4,1)-f(0,0,0)=40.

Question 5. Evaluate

\oint_{C} (x^{5}-y^{5}) dx + (x^{5}+y^{5}) dy,

where C denotes the boundary with positive orientation of the region between the circles x^{2}+y^{2}=a^{2} and x^{2}+y^{2}=b^{2} with 0<a<b.

Solution.

Method (i). The definition of the line integral.

On circle x^{2}+y^{2}=b^{2}, it is counterclockwise, x= b \cos \theta \text{ and } y= b \sin \theta,  \theta is from 0 to 2\pi.

On circle x^{2}+y^{2}=a^{2}, it is clockwise, x=a \cos \theta \text{ and } y= a \sin \theta, \theta is from 2\pi to 0.

On the circle C_{b}: x^{2}+y^{2}=b^{2} ,

\oint_{C_{b}} (x^{5}-y^{5})dx + ( x^{5}+y^{5}) dy

= b^{6} \int_{0}^{2\pi} ( \cos^{5} \theta - \sin^{5} \theta) \cdot ( - \sin \theta) + ( \cos^{5} \theta + \sin^{5} \theta) \cdot \cos \theta ) d \theta

= b^{6} \int_{0}^{2\pi} ( \cos^{6} \theta + \sin^{6} \theta) - \sin \theta \cos \theta ( \cos^{4} \theta - \sin^{4}\theta ) d\theta

= b^{6} \int_{0}^{2\pi} ( 1- 3\sin^{2} \theta \cos^{2}\theta - \frac{1}{2} \sin 2\theta \cos 2\theta ) d\theta

= b^{6} \int_{0}^{2\pi} ( \frac{5}{8} -\frac{3}{8} \cos 4\theta -\frac{1}{4} \sin 4 \theta ) d\theta

= b^{6} \cdot \frac{5}{4} \cdot \pi.

Pay attention to the orientation, we get the answer is

\frac{5 \pi}{4} ( b^{6}-a^{6}).

Method (ii). Green’s Theorem.

\oint_{C} (x^{5}-y^{5}) dx + (x^{5}+y^{5}) dy,

= \iint_{D}( 5 x^{4}+ 5 y^{4}) dxdy

= 5 \int_{0}^{2\pi} \int_{a}^{b} r^{5}( \cos^{4} \theta + \sin^{4} \theta) dr d \theta

= 5 \int_{a}^{b} r^{5} dr \cdot \int_{0}^{2 \pi} ( \cos^{4} \theta + \sin^{4}\theta) d\theta

= \frac{5( b^{6}-a^{6})}{6} \int_{0}^{2\pi} (1-2\sin^{2}\theta \cos^{2} \theta) d\theta

= \frac{5(b^{6}-a^{6})}{6} \cdot \frac{3 \pi}{2}

= \frac{5}{4} \pi ( b^{6}-a^{6}).

MA 1505 Mathematics I

MA 1505 Tutorial 8: Surface Area and Volume

Leave a comment

Assume z=z(x,y) is a surface on \mathbb{R}^{3}, the domain R is the projection of the surface z=z(x,y)  on xy-plane. Then the area of the surface is

\iint_{R} \sqrt{1+z_{x}^{2}+z_{y}^{2}} dxdy,

where z_{x} and z_{y} are partial derivatives of z=z(x,y) with respect to the variable x and y respectively.

If a surface is z=z(x,y)\geq 0 and the projection of it on xy-plane is R, then the volume bounded by xy-plane and the surface z=z(x,y) is

\iint_{R} z(x,y) dxdy.

Theorem 1. 

The surface area of the sphere with radius R is 4\pi R^{2}.

The volume of the sphere with radius R is \frac{4}{3} \pi R^{3}.

Proof.  The equation of the sphere with radius R is x^{2}+y^{2}+z^{2}=R^{2}.

First we calculate the surface area of sphere.

Assume R=\{ (x,y): x^{2}+y^{2} \leq R^{2} \}, the function z=z(x,y)=\sqrt{ R^{2}-x^{2}-y^{2}}.

Then

z_{x}=(-x)/ \sqrt{R^{2}-x^{2}-y^{2}},

z_{y}=(-y)/ \sqrt{R^{2}-x^{2}-y^{2}}.

Therefore

\sqrt{1+z_{x}^{2}+z_{y}^{2}} =R/ \sqrt{R^{2}-x^{2}-y^{2}}.

The surface area of half-sphere is

\iint_{x^{2}+y^{2}\leq R^{2}} \frac{R}{\sqrt{R^{2}-x^{2}-y^{2}}} dxdy

= \int_{0}^{2\pi} \int_{0}^{R} \frac{Rr}{\sqrt{ R^{2}-r^{2}}} dr d\theta

= 2 \pi R \int_{0}^{R} \frac{r}{\sqrt{R^{2}-r^{2}}} dr

= 2 \pi R^{2}

Hence, the total surface area of the sphere with radius R is 4\pi R^{2}.

Second, we calculate the volume of the sphere with radius R.

V= 2\iint_{x^{2}+y^{2} \leq R^{2}} \sqrt{R^{2}-x^{2}-y^{2}} dx dy

= 2 \int_{0}^{2\pi} \int_{0}^{R} \sqrt{R^{2}-r^{2}}\cdot r dr d\theta

= 2 \cdot 2\pi \cdot \int_{0}^{R} \sqrt{R^{2}-r^{2}}\cdot r dr

= \frac{4}{3} \pi R^{3}.

Theorem 2. The volume of the ellipsoid \frac{x^{2}}{a^{2}} +\frac{y^{2}}{b^{2}} + \frac{z^{2}}{c^{2}}=1 is \frac{4}{3}\pi abc.

Proof.

The upper bound of the volume is

z= c\cdot \sqrt{1-\frac{x^{2}}{a^{2}}- \frac{y^{2}}{b^{2}}}.

The lower bound of the volume is

z=- c\cdot \sqrt{1-\frac{x^{2}}{a^{2}}- \frac{y^{2}}{b^{2}}}.

Assume R=\{\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}} \leq 1 \}, the volume of the ellipsoid is

V= 2 \iint_{R} c \cdot \sqrt{1-\frac{x^{2}}{a^{2}}- \frac{y^{2}}{b^{2}}} dxdy

= 2 c \int_{0}^{2\pi} \int_{0}^{1} \sqrt{1-r^{2}} \cdot (r \cdot a \cdot b) dr d\theta

where we use the substitution x=a \cdot r \cos \theta and y=b\cdot r \sin \theta, the determinant of Jacobian matrix is a\cdot b\cdot r.

Therefore, the value equals to

2abc \cdot (2\pi) \int_{0}^{1} \sqrt{1-r^{2}} r dr = \frac{4}{3} \pi abc.

MA 1505 Mathematics I

MA 1505 Tutorial 2: Integration

Leave a comment

L.Hospital Rule: if the ratio is \infty/\infty or 0/0, then we can use the L.Hospital Rule to calculate the limit. Precisely, the L.Hospital Rule is 

\lim_{x\rightarrow a} \frac{f(x)}{g(x)}=\lim_{x\rightarrow a} \frac{f^{'}(x)}{g^{'}(x)},

where a is a finite real number or infinity.

If f(x) is a continuous function, then F(x)= \int_{a}^{x} f(t) dt is a differentiable function and its derivative F^{'}(x)=f(x).

General Leibniz Integration Rule.

\frac{d}{d\theta} ( \int_{a(\theta)}^{b(\theta)} f(x,\theta)dx)

= \int_{a(\theta)}^{b(\theta)} f_{\theta}(x,\theta) dx + f(b(\theta), \theta)\cdot b^{'}(\theta) - f(a(\theta),\theta) \cdot a^{'}(\theta)

Question 1. Calculate the value S=\int_{0}^{\frac{\pi}{2}} \frac{\cos x}{\sin x+\cos x}dx .

Solution. 

S=\int_{0}^{\frac{\pi}{2}} \frac{\cos x}{\sin x +\cos x }dx

= \int_{0}^{\frac{\pi}{2}} \frac{\cos(\frac{\pi}{2}-t)}{\sin(\frac{\pi}{2}-t) + \cos( \frac{\pi}{2}-t)} dt

= \int_{0}^{\frac{\pi}{2}} \frac{\sin t}{\cos t+\sin t}dt

Therefore

2S=S+S

= \int_{0}^{\frac{\pi}{2}} \frac{\cos x}{\sin x+\cos x} + \frac{\sin x}{\cos x+\sin x} dx

= \int_{0}^{\frac{\pi}{2}} 1 dx= \frac{\pi}{2}

Hence, S=\frac{\pi}{4} .

Question 2. Calculate the value S=\int_{0}^{\frac{\pi}{4}} \ln(1+\tan{x}) dx .

Solution. 

S=\int_{0}^{\frac{\pi}{4}} \ln(1+\tan x) dx

= \int_{0}^{\frac{\pi}{4}} \ln(1+\tan(\frac{\pi}{4}-x)) dx

= \int_{0}^{\frac{\pi}{4}} \ln(\frac{2}{1+\tan x})dx

= \frac{\pi \ln2}{4} - \int_{0}^{\frac{\pi}{4}} \ln(1+\tan x) dx

= \frac{\pi \ln2}{4} - S

Therefore, S=\frac{\pi \ln2}{8} .

Question 3. 

S=\int_{0}^{1} \frac{x^{4}}{x^{4}+(1-x)^{4}}dx

Solution.

S=\int_{0}^{1} \frac{x^{4}}{x^{4}+(1-x)^{4}}dx

= \int_{0}^{1} \frac{(1-x)^{4}}{x^{4}+(1-x)^{4}}dx

= 1- \int_{0}^{1} \frac{x^{4}}{x^{4}+(1-x)^{4}}dx

Therefore, S=0.5.

Question 4. 

\lim_{x\rightarrow 0} \frac{\sin x}{x}=1.

\lim_{x\rightarrow \infty} x\tan\frac{1}{x} = \lim_{y\rightarrow 0}\frac{\tan y}{y}=1, where y=1/x.

\lim_{x\rightarrow \infty} \frac{\ln x}{x^{a}}=0, where a>0.

MA 1505 Mathematics I

MA 1505 Tutorial 3: Taylor Series

Leave a comment

The Taylor Series of f(x) at the point x_{0} is

f(x)=\sum_{n=0}^{\infty} \frac{f^{(n)}(x_{0})}{n!} (x-x_{0})^{n}.

e^{x}=\sum_{n=0}^{\infty} \frac{x^{n}}{n!}

\ln(1+x)=\sum_{n=1}^{\infty} \frac{(-1)^{n-1}x^{n}}{n}

\sin x= \sum_{n=1}^{\infty} \frac{(-1)^{n-1} x^{2n-1}}{(2n-1)!}

\cos x =\sum_{n=0}^{\infty} \frac{(-1)^{n} x^{2n}}{(2n)!}

\frac{1}{1-x} =\sum_{n=0}^{\infty} x^{n}

Question 1. Let S=\sum_{n=0}^{\infty} \frac{1}{n!(n+2)} . Calculate the value of S.

Solution.

Method (i).

S=\sum_{n=0}^{\infty} \frac{1}{n!(n+2)}

= \sum_{n=0}^{\infty} \frac{n+1}{(n+2)!}

= \sum_{n=0}^{\infty} \frac{(n+2)-1}{(n+2)!}

= \sum_{n=0}^{\infty} (\frac{1}{(n+1)!}-\frac{1}{(n+2)!})

= 1

Method (ii). Integrate the Taylor series of xe^{x} to show that S=1.

The Taylor series of x e^{x} is \sum_{n=0}^{\infty} \frac{x^{n+1}}{n!} . Take the integration of the function on the interval [0,1], we get

\int_{0}^{1} xe^{x} dx

=\int_{0}^{1} \sum_{n=0}^{\infty} \frac{x^{n+1}}{n!} dx

= \sum_{n=0}^{\infty} \int_{0}^{\infty} \frac{x^{n+1}}{n!} dx

= \sum_{n=0}^{\infty} \frac{1}{n!(n+2)}=S .

The left hand side equals to 1 from integration by parts.

Method (iii). Differentiate the Taylor series of (e^{x}-1)/x.

The Taylor series of f(x)= (e^{x}-1)/x is \sum_{n=1}^{\infty} \frac{x^{n-1}}{n!} . Differentiate f(x) and get f^{'}(x)= \sum_{n=2}^{\infty} \frac{x^{n-2}}{(n-2)!n} . Moreover, f^{'}(x)= \frac{e^{x}x-(e^{x}-1)}{x^{2}} and f^{'}(1)=1=S.

Method (iv). Assume the function f(x)=\sum_{n=0}^{\infty} x^{n}/(n!(n+2)). This implies f(0)=0. Assume

g(x)=\int_{0}^{x}f(t)dt= \sum_{n=0}^{\infty} \int_{0}^{x} \frac{t^{n}}{n!(n+2)}dt = \sum_{n=0}^{\infty} \frac{x^{n+1}}{(n+2)!} = \frac{1}{x} \sum_{n=0}^{\infty} \frac{x^{n+2}}{(n+2)!} = \frac{1}{x}(e^{x}-1-x).

Since f(x)=g^{'}(x), we get f(x) = x^{-1}(e^{x}-1)-x^{-2}(e^{x}-1-x). That means f(1)=1.

Method (v). Assume the function f(x)=\sum_{n=0}^{\infty} x^{n}/(n!(n+2)).

f(x)= \sum_{n=0}^{\infty} \frac{x^{n}}{(n+1)!} - \frac{x^{n}}{(n+2)!} = x^{-1}\sum_{n=0}^{\infty} \frac{x^{n+1}}{(n+1)!} - x^{-2}\sum_{n=0}^{\infty} \frac{x^{n+2}}{(n+2)!} = x^{-1} (e^{x}-1) - x^{-2}(e^{x}-1-x). Therefore, f(1)=1.

Remark. There is a similar problem: calculate \sum_{n=0}^{\infty} \frac{(-1)^{n}}{n!(n+2)}. Answer is 1-2e^{-1}.

Question 2. Let n be a positive integer. Prove that

\frac{1}{2} \int_{0}^{1} t^{n-1}(1-t)^{2} dt= \frac{1}{n(n+1)(n+2)}

and calculate the value of the summation

S=\frac{1}{1\cdot 2 \cdot 3} + \frac{1}{3\cdot 4 \cdot 5} + \frac{1}{5\cdot 6\cdot 7} + \frac{1}{7\cdot 8 \cdot 9}+.... .

Solution. 

\frac{1}{2}\int_{0}^{1} t^{n-1}(1-t)^{2}dt

= \frac{1}{2} \int_{0}^{1} (t^{n+1}-2t^{n}+t^{n-1}) dt

= \frac{1}{2} (\frac{1}{n+2}-\frac{2}{n+1}+\frac{1}{n})

= \frac{1}{n(n+1)(n+2)} .

To calculate the value of S, there are two methods.

Method (i). The summation of S, n is only taken odd numbers. From the first step, we know the summation

S=\frac{1}{2} \int_{0}^{1} (1+t^{2}+t^{4}+t^{6}+...)(1-t)^{2}dt

= \frac{1}{2} \int_{0}^{1} \frac{1}{1-t^{2}} (1-t)^{2} dt

= \frac{1}{2} \int_{0}^{1} \frac{1-t}{1+t} dt

= \frac{1}{2} \int_{0}^{1} (\frac{2}{1+t}-1)dt

= \frac{1}{2}( 2\ln(1+t)-t)_{t=0}^{t=1}

= \ln 2 -\frac{1}{2} .

Method (ii).

Since \frac{1}{n(n+1)(n+2)}= \frac{1}{2}(\frac{1}{n}-\frac{2}{n+1}+\frac{1}{n+2}) ,

S=\sum_{ odd} \frac{1}{n(n+1)(n+2)}

= \frac{1}{2} \sum_{odd} ( \frac{1}{n}- \frac{2}{n+1}+\frac{1}{n+2})

= \frac{1}{2} ( \frac{1}{1}-\frac{2}{2}+\frac{1}{3}+ \frac{1}{3}-\frac{2}{4}+\frac{1}{5}+ \frac{1}{5}-\frac{2}{6}+\frac{1}{7}+\frac{1}{7}-\frac{2}{8}+\frac{1}{9}+...)

= \frac{1}{2} ( \frac{1}{1} + 2( -\frac{1}{2}+\frac{1}{3}-\frac{1}{4}+\frac{1}{5}-\frac{1}{6}+\frac{1}{7}-...))

= \frac{1}{2} ( 1 + 2 (\ln 2-1))

= \ln 2 -\frac{1}{2} .

Here we use the Taylor series of \ln(1+x)= \sum_{n=1}^{\infty} \frac{(-1)^{n-1}x^{n}}{n} and \ln 2= 1-\frac{1}{2}+\frac{1}{3}-\frac{1}{4}+\frac{1}{5}-\frac{1}{6}+....

Question 3. Assume \zeta(k)=1+\frac{1}{2^{k}} + \frac{1}{3^{k}} + ... = \sum_{m=1}^{\infty} \frac{1}{m^{k}}.

Prove

\sum_{k=2}^{\infty} (\zeta(k)-1)=1.

\sum_{k=1}^{\infty} (\zeta(2k)-1)=3/4.

Proof.

\sum_{k=2}^{\infty} (\zeta(k)-1)

= \sum_{k=2}^{\infty} \sum_{m=2}^{\infty} \frac{1}{m^{k}}

= \sum_{m=2}^{\infty} \sum_{k=2}^{\infty} \frac{1}{m^{k}}

= \sum_{m=2}^{\infty} \frac{1}{(m-1)m}

= \sum_{m=2}^{\infty} ( \frac{1}{m-1} - \frac{1}{m})

= 1.

\sum_{k=1}^{\infty} ( \zeta(2k)-1)

= \sum_{k=1}^{\infty} \sum_{m=2}^{\infty} \frac{1}{m^{2k}}

= \sum_{m=2}^{\infty} \sum_{k=1}^{\infty} \frac{1}{m^{2k}}

= \sum_{m=2}^{\infty} \frac{1}{m^{2}-1}

= \sum_{m=2}^{\infty} \frac{1}{2} ( \frac{1}{m-1}-\frac{1}{m+1})

= \frac{1}{2}(1+\frac{1}{2})

= \frac{3}{4}.

Question 4. Calculate the summation S= \sum_{k=1}^{\infty} \frac{(-1)^{k} k}{4k^{2}-1}.

Solution. 

S=\sum_{k=1}^{\infty} \frac{(-1)^{k} k}{4k^{2}-1}

=\frac{1}{4} \sum_{k=1}^{\infty} (-1)^{k} ( \frac{1}{2k-1} +\frac{1}{2k+1})

= \frac{1}{4} \sum_{k=1}^{\infty} ( \frac{(-1)^{k}}{2k-1} - \frac{(-1)^{k+1}}{2k+1})

= \frac{1}{4} \cdot \frac{-1}{2-1} = - \frac{1}{4}.

MA 1505 Mathematics I

MA 1505 Tutorial 7: Integration of Two Variables Functions

Leave a comment

In the tutorial 7, we will learn to calculate the integration of two variables, reverse the order of integration and polar coordinate.

The formulas of polar coordinate are x=r \cos(\theta), y=r \sin(\theta), where r\in (0,\infty) and \theta \in [0, 2\pi).

\iint_{D} f(x,y) dxdy= \iint_{D^{'}} f(r \cos \theta, r \sin \theta) r dr d\theta

Question 1. The application of polar coordinate. Calculate the value of

I= \int_{-\infty}^{\infty} e^{-x^{2}}dx.

Solution.

Method (i).

I=\int_{-\infty}^{\infty} e^{-x^{2}} dx= \int_{-\infty}^{\infty} e^{-y^{2}}dy .

Therefore

I^{2}=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} e^{-x^{2}-y^{2}} dxdy

= \int_{0}^{2\pi} \int_{0}^{\infty} e^{-r^{2}} r dr d\theta

= 2\pi \int_{0}^{\infty} e^{-r^{2}}r dr

= 2\pi \frac{1}{2} e^{-r^{2}}|_{r=0}^{r=\infty}

= \pi .

Hence I=\sqrt{\pi} .

Method (ii).

Since I=\int_{-\infty}^{\infty} e^{-x^{2}}dx = 2\int_{0}^{\infty} e^{-x^{2}}dx = 2\int_{0}^{\infty} e^{-y^{2}}dy , we get

I^{2}=\int_{0}^{\infty} \int_{0}^{\infty} e^{-x^{2}-y^{2}} dy dx

Assume y=sx, we get

I^{2}=4 \int_{0}^{\infty} \int_{0}^{\infty} e^{-x^{2}(1+s^{2})} x ds dx

=4 \int_{0}^{\infty} \int_{0}^{\infty} e^{-(1+s^{2})x^{2}} x dx ds

=4 \int_{0}^{\infty} \frac{1}{2(1+s^{2})} ds

=4 \cdot \frac{1}{2} \arctan s|_{s=0}^{s= \infty}

= \pi

Therefore, I=\sqrt{\pi}

Question 2. Calculate the value of

\lim_{t\rightarrow 0^{+}} \frac{\int_{0}^{t} \int_{x}^{t} \sin{y^{2}} dy dx}{t^{4}}.

Solution.

Method (i). Leibniz Integration Rule.

\frac{d}{d\theta} ( \int_{a(\theta)}^{b(\theta)} f(x,\theta)dx)

= \int_{a(\theta)}^{b(\theta)} f_{\theta}(x,\theta) dx + f(b(\theta), \theta)\cdot b^{'}(\theta) - f(a(\theta),\theta) \cdot a^{'}(\theta)

Here f_{\theta}(x,\theta) denotes the partial derivative of f(x, \theta) with respect to the variable \theta.

In the question, assume G(x,t)=\int_{x}^{t} \sin{y^{2}} dy .

Making use of L’Hospital Rule, we have

\lim_{t\rightarrow 0^{+}} \frac{\int_{0}^{t} \int_{x}^{t} \sin{y^{2}} dy dx}{t^{4}}

= \lim_{t\rightarrow 0^{+}} \frac{\int_{0}^{t} G(x,t)dx}{t^{4}}

= \lim_{t\rightarrow 0^{+}} \frac{\int_{0}^{t} G_{t}(x,t)dx+ G(t,t)\cdot 1 - G(0,t)\cdot 0}{ 4 t^{3}}

= \lim_{t\rightarrow 0^{+}} \frac{\int_{0}^{t} \sin{t^{2}}dx}{4t^{3}}

= \lim_{t\rightarrow 0^{+}} \frac{ t \sin{t^{2}}}{4t^{3}}= \frac{1}{4}

Method (ii). Reverse the order of integration.

The integration domain is 0\leq x \leq t and x \leq y \leq t. It is same as 0\leq y \leq t and 0\leq x\leq y.

Answer= \lim_{t\rightarrow 0^{+}} \frac{\int_{0}^{t} \int_{0}^{y} \sin{y^{2}} dxdy}{t^{4}}

= \lim_{t\rightarrow 0^{+}} \frac{\int_{0}^{t} y \sin{y^{2}}dy}{t^{4}}

= \lim_{t\rightarrow 0^{+}} \frac{ t \sin{t^{2}}}{4t^{3}}

=\frac{1}{4} .

Question 3. MA1505 2010-2011 Semester 2, Question 6(b).

Let R be a region of xy-plane, find the largest possible value of the integration

\iint_{R} (4-x^{2}-y^{2})dxdy.

Solution. 

Since we want to find the largest possible value, then we must guarantee that on the region R, the function f(x,y)=4-x^{2}-y^{2} is non-negative. That means the region R is 4-x^{2}-y^{2}\geq 0. i.e. x^{2}+y^{2}\leq 4. Therefore, we should calculate the integration

\iint_{x^{2}+y^{2}\leq 4} (4-x^{2}-y^{2}) dxdy

= \int_{0}^{2\pi} \int_{0}^{2} (4-r^{2})r dr d\theta

= 2\pi \int_{0}^{2} (4r-r^{3})dr

= 8\pi

Question 4. I \subseteq \mathbb{R} is a real interval, calculate the maximum value of

\int_{I} (1-x^{2}) dx.

Solution.

To calculate the maximum value of the integration, the maximal interval I=[-1,1]. Therefore, the maximum value of the integration is

\int_{-1}^{1} (1-x^{2}) dx = \frac{4}{3}.

Qustion 5. Calculate the multiple integration

\int_{0}^{1} \int_{0}^{\sqrt{1-x^{2}}} e^{x^{2}+y^{2}} dy dx.

Solution.

Method (i).  Use the polar coordinate.

\int_{0}^{1} \int_{0}^{\sqrt{1-x^{2}}} e^{x^{2}+y^{2}} dydx

= \int_{0}^{\pi/2} \int_{0}^{1} e^{r^{2}} r dr d\theta

= \frac{\pi}{2} \int_{0}^{1} e^{r^{2}} r dr

= \frac{\pi}{2} (\frac{e^{r^{2}}}{2}) |_{r=0}^{r=1}

= \frac{\pi}{4}(e-1).

Method (ii). Make the substitution y=sx, then dy=x ds.

The region is 0\leq x \leq 1 and 0\leq s \leq \sqrt{1-x^{2}}/x.

That is equivalent to 0 \leq s \leq \infty and 0 \leq x \leq 1/\sqrt{1+s^{2}}.

The integration is

\int_{0}^{1} \int_{0}^{\sqrt{1-x^{2}}/x} e^{x^{2}+s^{2}x^{2}} xds dx

= \int_{0}^{\infty} \int_{0}^{1/\sqrt{1+s^{2}}} e^{(1+s^{2})x^{2}} x dx ds

= \int_{0}^{\infty} (\frac{1}{2(1+s^{2})} e^{(1+s^{2})x^{2}} |_{x=0}^{x=1/\sqrt{1+s^{2}}}) ds

= \int_{0}^{\infty} \frac{e-1}{2(1+s^{2})}ds

= \frac{e-1}{2} \arctan s|_{s=0}^{s=\infty}

= \frac{\pi}{4} (e-1).

MA 1505 Mathematics I

MA 1505 Tutorial 6: Partial Derivatives and Directional Derivative

Leave a comment

In the tutorial, we will learn the partial derivatives for multiple variable functions.

Assume z=f(x,y) is a two variable function, then we use the notations to describe the partial derivatives of f(x,y).

f_{x}=\frac{\partial f}{\partial x} denotes the partial derivative of f under the variable x.

f_{y}=\frac{\partial f}{\partial y} denotes the partial derivative of f under the variable y.

Similarly, we can also define the second derivative of f(x,y).

f_{xx}=\frac{\partial^{2} f}{\partial x^{2}} ,

f_{xy}=f_{yx}=\frac{\partial^{2}f}{\partial x\partial y}=\frac{\partial^{2}f}{\partial y\partial x}  \text{ if } f(x,y) \text{ is a } C^{2} \text{ function.}

f_{yy}=\frac{\partial^{2} f}{\partial y^{2}} .

Assume u=(a,b) is a unit vector, i.e. its length is 1. If f(x,y) is C^{1} at the point p, then we can define the directional derivative of f(x,y) at point p as

f_{x}(p) a + f_{y}(p) b

Theorem 1. Geometric mean is not larger than Arithmetic mean.

For n positive real numbers a_{1}, a_{2}, ..., a_{n} ,

(a_{1}...a_{n})^{\frac{1}{n}} \leq \frac{a_{1}+...+a_{n}}{n}

“=” if and only if a_{1}=a_{2}=...=a_{n}.

Theorem 2. Cauchy’s Inequality.

For 2n real numbers a_{1},..., a_{n}, b_{1},...,b_{n} ,

(a_{1}b_{1}+...+a_{n}b_{n})^{2}\leq (a_{1}^{2}+...+a_{n}^{2})(b_{1}^{2}+...+b_{n}^{2})

“=” if and only if \frac{a_{1}}{b_{1}}=...=\frac{a_{n}}{b_{n}}.

Proof.

Method (i). Construct a non-negative function f(x) with respect to variable x

f(x)= \sum_{i=1}^{n}(a_{i}x-b_{i})^{2}= (\sum_{i=1}^{n} a_{i}^{2}) x^{2} - 2(\sum_{i=1}^{n} a_{i}b_{i}) x + (\sum_{i=1}^{n} b_{i}^{2}).

Consider the equation f(x)=0, there are only two possibilities: one is the equation f(x)=0 has only one root, the other one is the function has no real roots. Therefore,

\Delta=4(\sum_{i=1}^{n} a_{i}b_{i})^{2} - 4 ( \sum_{i=1}^{n} a_{i}^{2}) \cdot ( \sum_{i=1}^{n} b_{i}^{2}) \leq 0.

Hence, (a_{1}b_{1}+...+a_{n}b_{n})^{2}\leq (a_{1}^{2}+...+a_{n}^{2})(b_{1}^{2}+...+b_{n}^{2}).

Moreover, if “=”, then f(x)=0 has only one root x_{0}, i.e. for all 1\leq i \leq n, a_{i}x_{0}-b_{i}=0. That means

\frac{a_{1}}{b_{1}} =...=\frac{a_{n}}{b_{n}}.

By the way, the solution of ax^{2}+bx+c=0 is \frac{-b \pm \sqrt{b^{2}-4ac}}{2a} and \Delta=b^{2}-4ac.

Method (ii). Since a^{2}+b^{2}\geq 2 ab, we know

ab\leq \frac{1}{2}(\lambda^{2} a^{2}+ b^{2}/\lambda^{2}) for all \lambda \neq 0.

Assume \lambda^{2}=\sqrt{(\sum_{i=1}^{n}b_{i}^{2})/(\sum_{i=1}^{n} a_{i}^{2})} , for all 1\leq i \leq n,

a_{i}b_{i}\leq \frac{1}{2} (\lambda^{2}a_{i}^{2}+b_{i}^{2}/\lambda^{2})

Take the summation at the both sides,

\sum_{i=1}^{n} a_{i}b_{i} \leq \frac{1}{2}( \lambda^{2}\sum_{i=1}^{n}a_{i}^{2} + (\sum_{i=1}^{n} b_{i}^{2})/ \lambda^{2})= \sqrt{(\sum_{i=1}^{n} a_{i}^{2}) \cdot (\sum_{i=1}^{n}b_{i}^{2})}.

Question 1. Assume u(x,y) is a C^{2} function and u>0 . u(x,y) satisfies the partial differential equation u u_{xy}= u_{x}u_{y}.

Prove

(1) \frac{\partial \ln u}{\partial y} is a function of y.

(2) \frac{\partial \ln u}{\partial x} is a function of x.

(3) The solution of u(x,y) has the form u(x,y)=f(x) g(y) for some function f(x) and g(y) .

Proof.

(1) Method (i) Make use of derivative.

First, we know \frac{\partial \ln u}{\partial y}=\frac{u_{y}}{u} . Second, take the partial derivative of the function with respect to the variable x. That means,

\frac{\partial }{\partial x} (\frac{u_{y}}{u})= \frac{u_{xy}u- u_{x}u_{y}}{u^{2}}=0 from the partial differential equation. Therefore, the function \frac{u_{y}}{u} is independent of the variable x. i.e. the function is a function of variable y.

Method (ii) Make use of integration.

Since u u_{xy}=u_{x}u_{y} , \frac{u_{x}}{u}=\frac{u_{xy}}{u_{y}} , then we take the integration of x at the both sides,

\int \frac{u_{x}}{u} dx =\int \frac{u_{xy}}{u_{y}} dx , the left hand side is \ln u, the right hand side is \ln |u_{y}| + h_{1}(y) for some function h(y). That means, \frac{|u_{y}|}{u}=e^{-h_{1}(y)} . and \frac{u_{y}}{u} is a function of y .

(2) is similar to (1).

(3) From part (1), we know \frac{\partial \ln u }{\partial y} is a function of y. Assume \frac{\partial \ln u }{\partial y} = h_{2}(y). Take the integration of y at the both sides, we have

\ln u= \int h_{2}(y) dy + h_{3}(x) for some function h_{3}(x) . u = e^{\int h_{2}(y) dy} \cdot e^{h_{3}(x)} = g(y) \cdot f(x) for some functions f(x) and g(y).

Question 2. Assume L+K=150,  L and K are non-negative. Find the maximum value of f(L,K)=50 L^{0.4} K^{0.6} .

Solution.

Method (i). Langrange’s Method.

g(L,K,\lambda)=f(L,K)-\lambda(L+K-150)=50L^{\frac{2}{5}}K^{\frac{3}{5}}-\lambda(L+K-150).

Take three partial derivatives of g,

\frac{\partial g}{\partial \lambda} = -(L+K-150)=0

\frac{\partial g}{\partial L} = 50 \cdot \frac{2}{5} L^{-\frac{3}{5}}K^{\frac{3}{5}} - \lambda

\frac{\partial g}{\partial K}= 50 \cdot \frac{3}{5} L^{\frac{2}{5}} K^{-\frac{2}{5}} - \lambda=0

Solve these three equations, we get 2K=3L and L+K=150 , therefore the maximum value is taken at L=60 and K=90.

Method (ii). Change to one variable function.

Since L+K=150, we can define the one variable function

g(L)=f(L,150-L)=50 L^{\frac{2}{5}}(150-L)^{\frac{3}{5}}.

The derivative of g^{'}(L)=50 \cdot (\frac{2}{5} L^{-\frac{3}{5}}(150-L)^{\frac{3}{5}} - L^{\frac{2}{5}}\frac{3}{5}(150-L)^{-\frac{2}{5}}).

The critical point is L=60. The maximal value of g(L) is taken at L=60, K=90.

Method (iii). Mathematical Olympic Method.

Use the fact that the geometric mean is not larger than the arithmetic mean.

f(L,K)=50 L^{\frac{1}{5}}L^{\frac{1}{5}} K^{\frac{1}{5}} K^{\frac{1}{5}} K^{\frac{1}{5}}

= \frac{50}{3^{\frac{2}{5}} 2^{\frac{3}{5}}} (3L)^{\frac{1}{5}} (3L)^{\frac{1}{5}} (2K)^{\frac{1}{5}} (2K)^{\frac{1}{5}} (2K)^{\frac{1}{5}}

\leq \frac{50}{3^{\frac{3}{5}} 2^{\frac{2}{5}}} \frac{3L+3L+2K+2K+2K}{5}

= \frac{50}{3^{\frac{2}{5}} 2^{\frac{3}{5}}} \frac{6(L+K)}{5}

= \frac{50}{3^{\frac{2}{5}} 2^{\frac{3}{5}}} \frac{6\cdot 150}{5} .

The maximum value is taken at 3L=2K. i.e. L=60, K=90.

Question 3. Assume 24x+18y+12z=144 and x, y, z are non-negative variables. f(x,y,z)=18 x^{2} y z . Find the maximum value of f(x,y,z).

Solution.

Method (i). Langrange’s Method

g(x,y,z,\lambda)=f(x,y,z)-\lambda(24x+18y+12z-144) = 18 x^{2} y z-\lambda(24x+18y+12z-144)

Take four partial derivatives of g, the critical point is taken at x=z=1.5y. i.e. the maximum value of f(x,y,z) is taken at x=z=3, y=2.

Method (ii) Math Olympic Method

f(x,y,z)=18 x \cdot x \cdot y \cdot z

= \frac{18}{12*12*18*12} (12x) \cdot (12x) \cdot (18y) \cdot (12z)

\leq \frac{18}{12*12*18*12} (\frac{12x+12x+18y+12z}{4})^{4}

= \frac{18}{12*12*18*12} (\frac{144}{4})^{4}

The maximum value is taken at 12x=12x=18y=12z, i.e. x=z=3, y=2.

Question 4.  2012 Exam MA1505 Semester 1, Question 3(a)

Assume f(x,y) has continuous partial derivatives of all orders, if

\nabla f = (xy^{2}+kx^{2}y+x^{3}) \textbf{i} + (x^{3}+x^{2}y+y^{2}) \textbf{j},

Find the value of the constant k.

Solution.

Method (i) Use derivatives.

Since f has continuous partial derivative of all orders, f_{xy}= f_{yx}.

Since f_{x}= xy^{2}+kx^{2}y+x^{3} and f_{y}=x^{3}+x^{2}y+y^{2},

we have

\frac{\partial}{\partial y} (xy^{2}+kx^{2}y+x^{3})= \frac{\partial}{\partial x} (x^{3}+x^{2}y+y^{2})

This implies 2xy+kx^{2}=3x^{2}+2xy. i.e. k=3.

Method (ii). Use integration.

f_{x}=xy^{2}+kx^{2}y+x^{3} \Rightarrow f(x,y)=\frac{1}{2}x^{2}y^{2} + \frac{k}{3}x^{3}y + \frac{1}{4}x^{4} + h_{1}(y),

f_{y}=x^{3}+x^{2}y+y^{2} \Rightarrow f(x,y)=x^{3}y+\frac{1}{2}x^{2}y^{2}+\frac{1}{3}y^{3}+h_{2}(x).

Comparing them, we know k=3, h_{1}(y)=\frac{1}{3}y^{3}+C_{1} and h_{2}(x)=\frac{1}{4}x^{4}+C_{2}, where C_{1} and C_{2} are constants.

Therefore k=3.

MA 1505 Mathematics I

MA 1505 Tutorial 5: Fourier Series

2 Comments

In this tutorial, we will learn how to calculate the Fourier series of periodic functions.

Assume f(x) is a periodic function with period 2\pi, i.e. f(x)=f(x+2\pi) for all x \in \mathbb{R} . The Fourier Series of f(x) is defined as a_{0}+\sum_{n=1}^{\infty} (a_{n} \cos(nx) +b_{n} \sin(nx)), where

a_{0}= \frac{1}{2\pi} \int_{-\pi}^{\pi} f(x) dx,

a_{n}= \frac{1}{\pi} \int_{-\pi}^{\pi} f(x) \cos(nx) dx for all n\geq 1,

b_{n}= \frac{1}{\pi} \int_{-\pi}^{\pi} f(x) \sin(nx) dx for all n\geq 1,

Theorem 1.  If f(x) satisfies Lipchitz condition on (-\pi, \pi) , then

f(x) =a_{0}+\sum_{n=1}^{\infty} (a_{n} \cos(nx) +b_{n} \sin(nx)).

Theorem 2. Parseval’s Identity.

\frac{1}{\pi} \int_{-\pi}^{\pi} |f(x)|^{2} dx= 2a_{0}^{2}+ \sum_{n=1}^{\infty} (a_{n}^{2}+b_{n}^{2}).

Question 1. Assume f(x)=f(x+2\pi) for all x\in \mathbb{R} and f(x)=1505+1506x+1507x^{2}+1508x^{3} on [-\pi, \pi).

What is the value of a_{0}+\sum_{n=1}^{\infty}a_{n} ?

Solution. From Theorem 1, f(x)=a_{0}+\sum_{n=1}^{\infty} (a_{n} \cos(nx) +b_{n} \sin(nx)) on (-\pi, \pi) . Therefore, f(0)=a_{0}+\sum_{n=1}^{\infty}a_{n} and f(0)=1505 . Hence, a_{0}+\sum_{n=1}^{\infty}a_{n}=1505.

Question 2. Prove these identities:

\sum_{n=1}^{\infty} \frac{1}{(2n-1)^{2}}=\frac{\pi^{2}}{8}

\sum_{n=1}^{\infty} \frac{1}{n^{2}}=\frac{\pi^{2}}{6}

\sum_{n=1}^{\infty} \frac{1}{(2n-1)^{4}}=\frac{\pi^{4}}{96}

\sum_{n=1}^{\infty} \frac{1}{n^{4}}=\frac{\pi^{4}}{90}

Solution.

Choose the function f(x)=|x| on (-\pi, \pi) and f(x) is a periodic function with period 2\pi.

Use the formulas of a_{n} and b_{n}, we can prove that the Fourier series of f(x)=|x| is

\frac{\pi}{2} + \sum_{n=1}^{\infty} \frac{2((-1)^{n}-1)}{\pi} \cdot \frac{cos(nx)}{n^{2}}

From Theorem 1, take x=0, then

0= \frac{\pi}{2} + \sum_{n=1}^{\infty} \frac{2((-1)^{n}-1)}{n^{2} \pi} = \frac{\pi}{2} + \sum_{m=1}^{\infty} \frac{-4}{(2m-1)^{2}\pi} = \frac{\pi}{2} - \frac{4}{\pi} \sum_{m=1}^{\infty} \frac{1}{(2m-1)^{2}}

Therefore, \sum_{n=1}^{\infty} \frac{1}{(2n-1)^{2}}=\frac{\pi^{2}}{8} .

Assume S=\sum_{n=1}^{\infty} \frac{1}{n^{2}} , we get

S=\sum_{odd} \frac{1}{n^{2}} + \sum_{even} \frac{1}{n^{2}} = \frac{\pi^{2}}{8} + \frac{1}{4} S .

Therefore S=\frac{\pi^{2}}{6} .

From Parserval’s identity, we know

\frac{2\pi^{2}}{3}= \frac{1}{\pi} \int_{-\pi}^{\pi} x^{2}dx = 2\cdot (\frac{\pi}{2})^{2} + \sum_{n=1}^{\infty} \frac{4((-1)^{n}-1)^{2}}{\pi^{2}\cdot n^{4}} = \frac{\pi^{2}}{2} + \sum_{m=1}^{\infty} \frac{16}{\pi^{2} (2m-1)^{4}}

Therefore \sum_{n=1}^{\infty} \frac{1}{(2n-1)^{4}} = \frac{\pi^{4}}{96} .

Assume S=\sum_{n=1}^{\infty} \frac{1}{n^{4}} , we get

S=\sum_{odd} \frac{1}{n^{4}} + \sum_{even} \frac{1}{n^{4}} = \frac{\pi^{4}}{96} + \frac{1}{16} S

Therefore, S=\frac{\pi^{4}}{90} .